Tips and tricks for H2 A Level Pure Mathematics

Pure Mathematics can be very abstract. Often, the concepts that students have to learn are extremely theoretical and intellectual. There seems to be no beauty to its madness. As Singapore’s top JC tuition center, Zenith hopes to be able to break down the syllabus and impart key skills for each theme.

A Level Pure Math is notorious for being very challenging for many students. Being the portion that accrues most of your grade, it is important to score well because that could very well make or break your distinction! The H2 A Level Math paper is split into 2: Paper 1 consists entirely of Pure Math. For Paper 2, 40% of it consists of Pure Math questions. With each paper occupying half of the total grade, its total weightage is a whopping 70% of your final grade!

Our JC Math tuition programme classifies the various topics of H2 Pure Mathematics at the A Levels into five distinct themes (Fig 1). Each theme is unique and contains a few subtopics that are interrelated. In the following article, Zenith provides curated tips and tricks for each Pure Math theme, so that you can tackle them with courage and conviction!

Be strong in your foundations

Pure Mathematics chapters are highly vigorous with regards to the theoretical side of things. This means that a variety of theorems and concepts underpin a substantial number of Pure Math topics. This is especially pertinent for themes from Group 1 and Group 4, which are Functions & Graphs and Complex Numbers respectively. These groups rely on a strong foundational grounding in core mathematical concepts. For Group 1, a good grasp of Algebra is required for H2 A Level Math students to do well. For Group 4, a solid base of Trigonometry and Logarithms would help a ton!

At the best Math tuition in Singapore, we break down the question above as asking for three Algebraic equations. Despite the lengthiness of context, it aims to test students on their grasp of basic Algebraic concepts.

All that is required is for you to methodologically convert the wordy statements into three distinct Algebraic equations! Equation 2 might be a bit complex, but it is essentially the addition of interest from x and z with doubly compounded interest from y (hence the 1.032 – 1). Once you are able to derive all three, simply plug them into your graphing calculator to solve for the answer.

Apart from Algebraic equations, Algebra also permeates the chapters of Graphs and Functions, which are under Group 1 as well. You may be called on to find the equations of asymptotes or the effect of transformations on graphs. All of which require you to be adept at manipulating Algebraic statements! Similarly, finding the domains, ranges, and inverses of functions cannot be achieved without you being able to effortlessly wield Algebraic equations in your hands! For the theme that is Group 1, having a solid grounding in Algebra is absolutely necessary.

For the chapters under the umbrella of Group 4, Complex Numbers, the Trigonometry terms of Cosine and Sine are a staple whenever the polar form is required. Also, Complex Numbers in their polar form are often required to be represented on the four quadrants of a graph, or an Argand diagram. This implies that on top of having a solid base in Trigonometry, a strong foundation in Angles, for the argument of the Complex Number (z), is a necessity to be able to score well in this theme! Furthermore, a good grasp of Modulus and Pythagoras Theorem would do wonders in being able to interpret the distance (magnitude) of the complex number (z) from the origin.

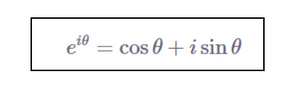
From the above, with the right-hand side of the equation representing the polar form of the Complex Number z (Trigo) as previously mentioned, the left-hand side thus denotes the exponential form of the Complex Number. Thus, this is where comprehension of Logarithms and their associated laws come into play when we need to manipulate the exponential form of a Complex Number.

Fine-tune your visualization skills

Being able to accurately visualize is a key skill that Zenith’s JC Math tuition programme seeks to instill in our students. This applies especially to Group 3, which encompasses basic Vectors all the way to complex 3-Dimensional Vector models. Often, a good degree of imagination is needed to visualize the representation of the diagrams in space and subsequently solve them.

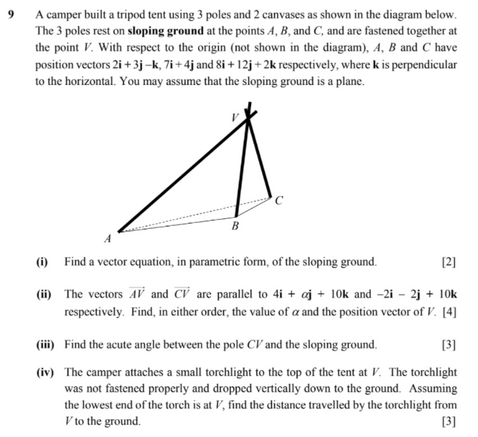
Fig 4 asks us to define the equation of a plane, an acute angle, and an unknown distance that is to be deduced from the intricacies of the given data. In this case, a pre-planned Vector model was provided so we’re able to get a better grasp on the demands of the question. However, at the H2 A Level Math examinations, the same cannot be said for the questions set, so it is entirely up to you to construct rough sketches for such visualization heavy questions! When it comes to the theme of Vectors, simply memorizing the formulas from your notes would not be enough; you need to actively understand their relationships in a visual form in order to be sufficiently prepared. If you are worried – don’t be! At the top Math tuition center in Singapore, Zenith develops your skills in the visualization arena to the best of our abilities. With our help, the topics of theme 3 will be a breeze!

Active Application

The last tip that Zenith recommends for H2 Pure Math is knowing how to apply all you have learnt situationally. This skill is needed especially for the topics under Groups 2 and 5. For these two themes, there is an abundance of formulas to familiarise oneself with. What is more important, however, is that you understand which formulas to select and how to tailor them to the demands of the question. With regard to Group 2, there are only two main sequential formulas to keep in mind, one for Geometric Progressions, and another for Arithmetic Progressions. Yet at the A Levels, pattern recognition questions are often phrased in novel ways with the aim of tripping up unwitting students (Fig 5.)

For (i), three simultaneous equations are actually required to be defined and solved with the aid of a graphing calculator. This shows how skills in the topic of Equations and Inequalities, from Group 1, for instance, can be applied to this topic of Sequences and Series. For (ii), the general ability to manipulate Algebraic functions ably is paramount in addressing the requirements of the Geometric Series. Hence, we see the interchangeability and interdisciplinary nature of such topics, where separate themes are often fitted in conjunction with other themes. You’ll need to be able to see through the smokescreen of unfamiliar question types and apply formulas not only relevant to that theme, but also from other chapters.

Calculus (Group 5) is slightly different. Our team at the best Math tuition in Singapore has identified it to be the most formula-heavy in nature. What this means is that there are a plethora of formulas to be acquainted with. Yet, knowing how to use your knowledge of the various differential and integral methods is key. While this theme may be highly theoretical, the questions mostly come with a context. This is because many real-life problems are modeled upon the realm of Calculus. Sometimes, Calculus questions may require that you sketch out a graph. With regards to the theme of Differentiation and Integration, concepts from Trigonometry are also highly intertwined with them! This once again shows how the topics are tightly tied together with each other.

With the above tips in mind, Zenith is confident that you can go forth to ace the Pure Math sections of your JC H2 Math Papers! As the top Math tuition in Singapore, Zenith provides you with all of the resources required to do well. We always make sure to nurture the mathematical side of each student so that they can tackle their H2 A Level Math exams with confidence! Well, what are you waiting for? Click here to be a part of Zenith’s JC Math tuition program! You may also visit our testimonials page to find out more or contact us for a free trial today!